1
2
3
4
5
6
7
8
9
10
11
12
13
14
15
16
17
18
19
20
21
22
23
24
25
26
27
28
29
30
31
32
33
34
35
36
37
38
39
40
41
42
43
44
45
46
47
48
49
50
51
52
53
54
55
56
57
58
59
60
61
62
63
64
65
66
67
68
69
70
71
72
73
74
75
76
77
78
79
80
81
82
83
84
85
86
87
88
89
90
91
92
93
94
95
96
97
98
99
100
101
102
103
104
105
106
107
108
109
110
111
112
113
114
115
116
117
118
119
120
121
122
123
124
125
126
127
128
129
130
131
132
133
134
135
136
137
138
139
140
141
142
143
144
145
146
147
148
149
150
151
152
153
154
155
156
157
158
159
160
161
162
163
164
165
166
167
168
| #include<bits/stdc++.h>
using namespace std;
#define ll long long
const int N = 500010;
int n, k;
struct Point
{
int x[2], w;
Point() {};
Point(int _x, int _y, int _w)
{
x[0] = _x, x[1] = _y, w = _w;
}
friend bool operator == (const Point &a, const Point &b)
{
return (a.x[0] == b.x[0]) && (a.x[1] == b.x[1]);
}
};
const double alpha = 0.75;
struct KDT
{
int ls, rs;
int l[2], r[2];
int sum, sz;
Point p;
}tr[N];
int rt, idx = 0;
int rec[N], tt;
int newnode()
{
if(tt)return rec[tt--];
else return ++idx;
}
void pushup(int p)
{
tr[p].l[0] = tr[p].r[0] = tr[p].p.x[0];
tr[p].l[1] = tr[p].r[1] = tr[p].p.x[1];
tr[p].sum = tr[p].p.w;
tr[p].sz = 1;
if(tr[p].ls)
{
tr[p].l[0] = min(tr[p].l[0], tr[tr[p].ls].l[0]);
tr[p].l[1] = min(tr[p].l[1], tr[tr[p].ls].l[1]);
tr[p].r[0] = max(tr[p].r[0], tr[tr[p].ls].r[0]);
tr[p].r[1] = max(tr[p].r[1], tr[tr[p].ls].r[1]);
tr[p].sum += tr[tr[p].ls].sum;
tr[p].sz += tr[tr[p].ls].sz;
}
if(tr[p].rs)
{
tr[p].l[0] = min(tr[p].l[0], tr[tr[p].rs].l[0]);
tr[p].l[1] = min(tr[p].l[1], tr[tr[p].rs].l[1]);
tr[p].r[0] = max(tr[p].r[0], tr[tr[p].rs].r[0]);
tr[p].r[1] = max(tr[p].r[1], tr[tr[p].rs].r[1]);
tr[p].sum += tr[tr[p].rs].sum;
tr[p].sz += tr[tr[p].rs].sz;
}
}
bool cmp0(Point a, Point b)
{
return a.x[0] < b.x[0];
}
bool cmp1(Point a, Point b)
{
return a.x[1] < b.x[1];
}
bool canrbu(int p)
{
return (1.0 * max(tr[tr[p].ls].sz, tr[tr[p].rs].sz)) >= (alpha * tr[p].sz);
}
Point ldr[N];
int ldc;
void rbuunf(int p)
{
if(!p)return;
rbuunf(tr[p].ls);
if(tr[p].p.w)
{
ldr[++ldc] = tr[p].p;
rec[++tt] = p;
}
rbuunf(tr[p].rs);
}
int rbubld(int l, int r, int k)
{
if(l > r)return 0;
int mid = (l + r) >> 1;
int p = newnode();
nth_element(ldr + l, ldr + mid, ldr + r + 1, k ? cmp1 : cmp0);
tr[p].p = ldr[mid];
tr[p].ls = rbubld(l, mid - 1, k ^ 1);
tr[p].rs = rbubld(mid + 1, r, k ^ 1);
pushup(p);
return p;
}
void rbuild(int &p, int k)
{
ldc = 0;
rbuunf(p);
p = rbubld(1, ldc, k);
}
void insert(int &p, Point v, int k)
{
if(!p)
{
p = newnode();
tr[p].ls = tr[p].rs = 0;
tr[p].p = v;
pushup(p);
return;
}
if(v.x[k] <= tr[p].p.x[k])insert(tr[p].ls, v, k ^ 1);
else insert(tr[p].rs, v, k ^ 1);
pushup(p);
if(canrbu(p))rbuild(p, k);
}
inline bool inc(Point p, int x1, int y1, int x2, int y2)
{
return (p.x[0] >= x1) && (p.x[0] <= x2) && (p.x[1] >= y1) && (p.x[1] <= y2);
}
inline bool inc(int p, int x1, int y1, int x2, int y2)
{
return (tr[p].l[0] >= x1) && (tr[p].r[0] <= x2) && (tr[p].l[1] >= y1) && (tr[p].r[1] <= y2);
}
inline bool exc(int p, int x1, int y1, int x2, int y2)
{
return (tr[p].l[0] > x2) || (tr[p].r[0] < x1) || (tr[p].l[1] > y2) || (tr[p].r[1] < y1);
}
int query(int p, int x1, int y1, int x2, int y2)
{
if(!p)return 0;
if(exc(p, x1, y1, x2, y2))return 0;
if(inc(p, x1, y1, x2, y2))return tr[p].sum;
int res = 0;
if(inc(tr[p].p, x1, y1, x2, y2))res += tr[p].p.w;
res += query(tr[p].ls, x1, y1, x2, y2);
res += query(tr[p].rs, x1, y1, x2, y2);
return res;
}
int main()
{
scanf("%d", &n);
int lastans = 0, op;
while(scanf("%d", &op), op != 3)
{
if(op == 1)
{
int x, y, w;
scanf("%d%d%d", &x, &y, &w);
x ^= lastans, y ^= lastans, w ^= lastans;
insert(rt, Point(x, y, w), 0);
}
else if(op == 2)
{
int x1, y1, x2, y2;
scanf("%d%d%d%d", &x1, &y1, &x2, &y2);
x1 ^= lastans, y1 ^= lastans, x2 ^= lastans, y2 ^= lastans;
lastans = query(rt, x1, y1, x2, y2);
printf("%d\n", lastans);
}
}
}
|

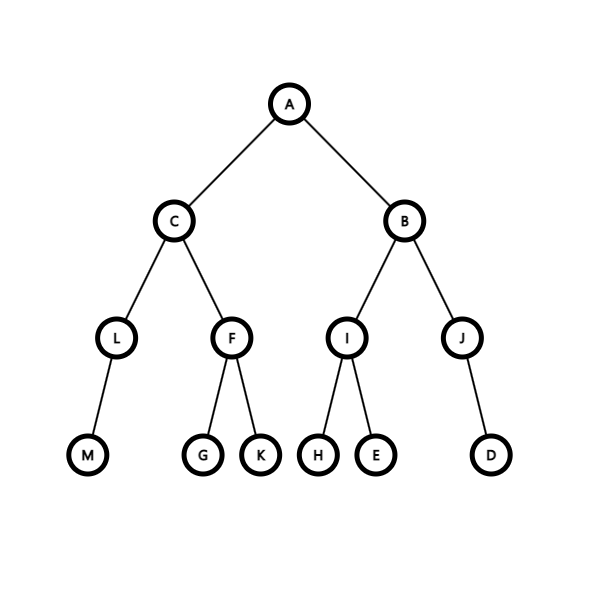



未找到相关的 Issues 进行评论
请联系 @KaiserWilheim 初始化创建